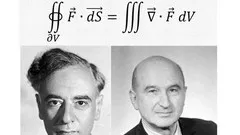
Multivariable Calculus and Classical Physics problems

Course Overview

What does this course tell? (Please note that the following overview content is from the original platform) In the first part of this course Multivariable Calculus is explained by focusing on understanding the key concepts rather than learning the formulas and&or exercises by rote The process of reasoning by using mathematics is the primary objective of the course and not simply being able to do computations Besides interesting proofs will be given such as the Gauss and Stokes theorems proofsThe prior knowledge requirement is Single variable Calculus (even without a great mastery of it)I will list some of the most important concepts that we will see here in the followingpartial differentiation The partial derivative generalizes the notion of the derivative to higher dimensions A partial derivative of a multivariable function is a derivative with respect to one variable with all other variables held constant Partial derivatives may be combined in interesting ways to create more complicated expressions of the derivative For example in vector calculus (which we will see) the "del" operator is used to define the concepts of gradient divergence and curl in terms of partial derivatives A matrix of partial derivatives the Jacobian matrix may be used to represent the derivative of a function between two spaces of arbitrary dimension Differential equations containing partial derivatives are called partial differential equations or PDEs These equations are generally more difficult to solve than ordinary differential equations which contain derivatives with respect to only one variable (PDEs are not discussed in this course)Multiple integration The multiple integral extends the concept of the integral to functions of any number of variables Double and triple integrals may be used to calculate areas and volumes of regions in the plane and in space The surface integral and the line integral are used to integrate over curved manifolds such as surfaces and curves We will see these conceptsI am available for questions which I could answer by (possibly) uploading new content to the course namely videos containing the solutionThe second part of this course is about solving advanced mechanics problems; since multivariable calculus is a staple of this second part I decided to combine the part on physics problems and the one on multivariable calculus into a single course where you can therefore find lots of material This set of problems is taken from the first volume of the course of theoretical physics by Landau and Lifshitz I have selected some problems from this book and provided a thorough step-by-step solution in the course; the solutions to these problems are also given in the book but they are usually quite terse namely not many details are provided Therefore what we will do in the course is to first construct the necessary theory to deal with the problems and then we will solve the problems Some theory is also discussed while solving the problems themselves Every single formula in this course is motivated&derivedWe will start from the action principle whose main constituent is the Lagrangian which is fundamental to dealing with advanced problems in all branches of physics even if we restrict ourselves to mechanics in this case We will solve several problems related to how to construct a Lagrangian of a (possibly complex) system and we will also derive the Hamiltonian from the Lagrangian which represents the energy of a system and do some problems on thatWe will also study the kinematics of rigid bodies and derive formulae for the velocities of points which belong to the bodies as well as formulae for accelerations Accelerations are important not just for kinematics but also for the dynamics of rigid bodiesAs regards the motion of rigid bodies we will discuss the kinetic energy which is necessary to obtain the Lagrangian and solve several problems in three dimensions related to how to find the kinetic energy of a body in motionThe expression of the kinetic energy is dependent on the angular velocity (which is a concept that we will derive in kinematics) and also depends on the inertia matrix (or inertia tensor) which we will also derive The formulae will be therefore written in a very general form and this is useful when tackling difficult problems since knowing a general method will provide the means to solve themThe inertia tensor will appear in the expression for the kinetic energy and it will also appear in dynamics in the formula for moments; we will see why it appears and use the theory to solve problemsWe will also discuss non-inertial frames and find the deflection of a freely falling body from the vertical caused by the Earths rotation (which makes the Earth a non-inertial frame) We considered the value of this course from many aspects, and finally summarized it for you from two aspects: skills and knowledge, and the people who benefit from it: (Please note that our content is optimized through artificial intelligence tools and carefully reviewed by our editorial staff.) What skills and knowledge will you acquire during this course? During this course on Multivariable Calculus and Classical Physics problems, students will acquire the following skills and knowledge: 1. Understanding of partial differentiation: Students will learn how to compute partial derivatives of multivariable functions and understand their geometric interpretation. They will also learn how to combine partial derivatives to create more complex expressions of the derivative. 2. Knowledge of vector calculus: Students will study vector calculus concepts such as gradient, divergence, and curl, which are defined in terms of partial derivatives. They will also learn about the "del" operator and its applications in vector calculus. 3. Familiarity with the Jacobian matrix: Students will learn how to use the Jacobian matrix to represent the derivative of a function between two spaces of arbitrary dimension. 4. Introduction to multiple integration: Students will extend their understanding of integration to functions of multiple variables. They will learn how to compute double and triple integrals to calculate areas and volumes of regions in the plane and in space. 5. Understanding of surface and line integrals: Students will learn how to integrate over curved manifolds such as surfaces and curves using surface and line integrals. 6. Ability to solve advanced mechanics problems: Students will develop problem-solving skills in advanced mechanics by applying the concepts of multivariable calculus. They will learn how to construct Lagrangians for complex systems and derive the Hamiltonian from the Lagrangian. 7. Knowledge of kinematics and dynamics of rigid bodies: Students will study the kinematics of rigid bodies, including the derivation of formulas for velocities and accelerations of points on the bodies. They will also learn about the concept of angular velocity and its role in the expression of kinetic energy. 8. Understanding of inertia tensor: Students will learn about the inertia tensor and its significance in the expression of kinetic energy and moments in dynamics. They will understand how to use the inertia tensor to solve problems related to rigid body motion. 9. Familiarity with non-inertial frames: Students will study non-inertial frames and their effects on the motion of objects. They will learn how to calculate the deflection of a freely falling body caused by the Earth's rotation. Overall, this course will provide students with a strong foundation in multivariable calculus and its applications in classical physics problems. They will develop problem-solving skills and gain a deeper understanding of the mathematical concepts underlying these problems. Who will benefit from this course? This course on Multivariable Calculus and Classical Physics problems will benefit individuals who have a background in single variable calculus and are interested in understanding key concepts rather than memorizing formulas and exercises. It is particularly useful for those pursuing careers or interests in physics, engineering, mathematics, or any field that requires a strong foundation in calculus and physics. Specific professions that may benefit from this course include: 1. Physicists: The course covers advanced mechanics problems and provides a thorough understanding of concepts such as the Lagrangian, Hamiltonian, and kinematics of rigid bodies. This knowledge is essential for physicists working in various branches of physics. 2. Engineers: Multivariable calculus is widely used in engineering fields, and this course provides a comprehensive understanding of partial differentiation, multiple integration, and their applications in solving problems related to areas, volumes, surfaces, and curves. The course also covers topics such as non-inertial frames, which are relevant in engineering applications. 3. Mathematicians: The course delves into the mathematical foundations of multivariable calculus, including partial derivatives, Jacobian matrices, and differential equations. Mathematicians can benefit from the rigorous approach to reasoning and problem-solving presented in the course. 4. Students pursuing higher education: This course can serve as a valuable resource for students who are preparing for advanced courses in physics, engineering, or mathematics. It provides a solid understanding of fundamental concepts and problem-solving techniques that are essential for success in higher-level courses. Overall, anyone with an interest in deepening their understanding of multivariable calculus and its applications in classical physics will benefit from this course.